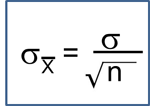Sample Size for One Sample, Dichotomous Outcome
In studies where the plan is to estimate the proportion of successes in a dichotomous outcome variable (yes/no) in a single population, the formula for determining sample size is:

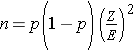
where Z is the value from the standard normal distribution reflecting the confidence level that will be used (e.g., Z = 1.96 for 95%) and E is the desired margin of error. p is the proportion of successes in the population. Here we are planning a study to generate a 95% confidence interval for the unknown population proportion, p. The equation to determine the sample size for determining p seems to require knowledge of p, but this is obviously this is a circular argument, because if we knew the proportion of successes in the population, then a study would not be necessary! What we really need is an approximate value of p or an anticipated value. The range of p is 0 to 1, and therefore the range of p(1-p) is 0 to 1. The value of p that maximizes p(1-p) is p=0.5. Consequently, if there is no information available to approximate p, then p=0.5 can be used to generate the most conservative, or largest, sample size.
Example 2:
An investigator wants to estimate the proportion of freshmen at his University who currently smoke cigarettes (i.e., the prevalence of smoking). How many freshmen should be involved in the study to ensure that a 95% confidence interval estimate of the proportion of freshmen who smoke is within 5% of the true proportion?
Because we have no information on the proportion of freshmen who smoke, we use 0.5 to estimate the sample size as follows:

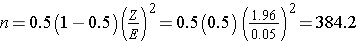
In order to ensure that the 95% confidence interval estimate of the proportion of freshmen who smoke is within 5% of the true proportion, a sample of size 385 is needed.

Suppose that a similar study was conducted 2 years ago and found that the prevalence of smoking was 27% among freshmen. If the investigator believes that this is a reasonable estimate of prevalence 2 years later, it can be used to plan the next study. Using this estimate of p, what sample size is needed (assuming that again a 95% confidence interval will be used and we want the same level of precision)?
Example 3:
An investigator wants to estimate the prevalence of breast cancer among women who are between 40 and 45 years of age living in Boston. How many women must be involved in the study to ensure that the estimate is precise? National data suggest that 1 in 235 women are diagnosed with breast cancer by age 40. This translates to a proportion of 0.0043 (0.43%) or a prevalence of 43 per 10,000 women. Suppose the investigator wants the estimate to be within 10 per 10,000 women with 95% confidence. The sample size is computed as follows:

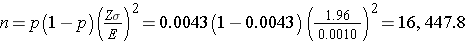
A sample of size n=16,448 will ensure that a 95% confidence interval estimate of the prevalence of breast cancer is within 0.10 (or to within 10 women per 10,000) of its true value. This is a situation where investigators might decide that a sample of this size is not feasible. Suppose that the investigators thought a sample of size 5,000 would be reasonable from a practical point of view. How precisely can we estimate the prevalence with a sample of size n=5,000? Recall that the confidence interval formula to estimate prevalence is:

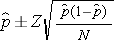 .
.
Assuming that the prevalence of breast cancer in the sample will be close to that based on national data, we would expect the margin of error to be approximately equal to the following:


Thus, with n=5,000 women, a 95% confidence interval would be expected to have a margin of error of 0.0018 (or 18 per 10,000). The investigators must decide if this would be sufficiently precise to answer the research question. Note that the above is based on the assumption that the prevalence of breast cancer in Boston is similar to that reported nationally. This may or may not be a reasonable assumption. In fact, it is the objective of the current study to estimate the prevalence in Boston. The research team, with input from clinical investigators and biostatisticians, must carefully evaluate the implications of selecting a sample of size n = 5,000, n = 16,448 or any size in between.