Risk Ratios and Rate Ratios (Relative Risk)
Measures of disease frequency can be compared by calculating their ratio. Common terms to describe these ratios are
- risk ratio
- rate ratio
- relative risk
- relative rate
Frequently, the term "relative risk" is used to encompass all of these. These relative measures give an indication of the "strength of association."
Risk Ratio
For the study examining wound infections after incidental appendectomy, the risk of wound infection in each exposure group is estimated from the cumulative incidence. The relative risk (or risk ratio) is an intuitive way to compare the risks for the two groups. Simply divide the cumulative incidence in exposed group by the cumulative incidence in the unexposed group:
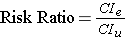
where CIe is the cumulative incidence in the 'exposed' group and CIu is the cumulative incidence in the 'unexposed' group.
The table below shows how the risk ratio was calculated in the study examining the risk of wound infections when an incidental appendectomy was done during a staging laparotomy for Hodgkin disease.
|
Had Incidental Appendectomy? |
Wound Infection |
No Wound Infection |
Total |
Cumulative Incidence |
|
Yes |
7 |
124 |
131 |
7/131 = 5.34% |
|
No |
1 |
78 |
79 |
1/79 = 1.27% |
Risk Ratio = 5.34/1.27 = 4.2
Organization of the information in a contingency table facilitates analysis and interpretation. The cumulative incidence is an estimate of risk. Incidental appendectomies were performed in a total of 131 patients, and seven of these developed post-operative wound infections, so the cumulative incidence was 7 divided by 131, or 5.34%. We can similarly calculate the cumulative incidence in the patients who did not have an incidental appendectomy, which was 1 divided by 79 or 1.27%. So, the risk ratio is 5.34/1.27 or 4.2.
Interpretation:
In this study patients who underwent incidental appendectomy had 4.2 times the risk of post-operative wound infection compared to patients who did not undergo incidental appendectomy.
Risk Ratio <1
It is also possible for the risk ratio to be less than 1; this would suggest that the exposure being considered is associated with a reduction in risk. In 1982 The Physicians' Health Study (a randomized clinical trial) was begun in order to test whether low-dose aspirin was beneficial in reducing myocardial infarctions (heart attacks). The study population consisted of over 22,000 male physicians who were randomly assigned to either low-dose aspirin or a placebo (an identical looking pill that was inert). They followed these physicians for about five years. Some of the data is summarized in the 2x2 table shown below.
|
Treatment |
Myocardial Infarction |
No Infarction |
Total |
Cumulative Incidence |
|
Aspirin |
139 |
10,898 |
11,037 |
139/11,037 = 0.0126 |
|
Placebo |
239 |
10,795 |
11,034 |
239/11,034 = 0.0217 |
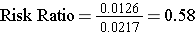
Note that the "exposure" of interest was low-dose aspirin, and the aspirin group is summarized in the top row. The group assigned to take aspirin had an incidence of 1.26%, while the placebo (unexposed) group had an incidence of about 2.17%. The cumulative incidence in the aspirin group was divided by the cumulative incidence in the placebo group, and RR= 0.58. An appropriate interpretation of this would be:
Those who take low dose aspirin regularly have 0.58 times the risk of myocardial infarction compared to those who do not take aspirin.
Note also that the unexposed (comparison, reference) group must be specified. For example, if we simply said, "Those who take low dose aspirin regularly have 0.58 times the risk of myocardial infarction", the question is "compared to what?" Is it those who didn't take any aspirin, those who took low-dose aspirin but used it irregularly, those who took high dose aspirin, those who took acetaminophen...?
In general:
- If the risk ratio is 1 (or close to 1), it suggests no difference or little difference in risk (incidence in each group is the same).
- A risk ratio > 1 suggests an increased risk of that outcome in the exposed group.
- A risk ratio < 1 suggests a reduced risk in the exposed group.
Percent Relative Effect
An alternative way to look at and interpret these comparisons would be to compute the percent relative effect (the percent change in the exposed group). In essence, we regard the unexposed group as having 100% of the risk and express the exposed group relative to that. For example,
When RR > 1
For the wound infection study, the group that had the incidental appendectomy had a 320% increase in risk over and above the risk in the unexposed group (100%). When RR > 1,
% increase = (RR - 1) x 100, e.g. (4.2 - 1) x 100 = 320% increase in risk.
Interpretation: Those who had the incidental appendectomy had a 320% increase in risk of getting a post-operative wound infection.
When RR < 1
For the aspirin study, the men on low-dose aspirin had a 43% reduction in risk. When RR < 1,
% decrease = (1 - RR) x 100, e.g. (1 - 0.57) x 100 = 43% decrease in risk
Interpretation: Those who took low-dose aspirin had a 43% reduction in risk of myocardial infarction compared to those who did not take aspirin.
Pitfalls: Note that in the interpretation of RR both the appendectomy study (in which the RR > 1), and the aspirin trial (in which RR < 1) used the expression "times the risk." To be precise, it is not correct to say that those who had an incidental appendectomy had 4.2 times more risk (wrong) or 4.2 times greater risk (wrong). In fact, those with the incidental appendectomy had a 320% increase in risk. Conversely, in the aspirin study it is not correct to say that those on aspirin had 0.57 times less risk (wrong). In fact, they had 43% less risk.
CORRECT:
- Subjects taking aspirin had 0.57 times the risk of myocardial infarction compared to subjects taking the placebo.
- The risk of myocardial infarction among subjects taking aspirin was 0.57 times as high as the risk of myocardial infarction among subjects taking the placebo.
- Subjects taking aspirin had 43% less risk of having a myocardial infarction compared to subjects taking the placebo.
- Subjects who underwent incidental appendectomy had 4.2 times the risk of wound infection compared to subjects who did not undergo appendectomy.
- Subjects who underwent incidental appendectomy were 4.2 times as likely to develop a wound infection compared to subjects who did not undergo appendectomy (i.e., the probability of the outcome in the exposed group was 4.2 times that of the unexposed group).
- The risk of wound infection who underwent incidental appendectomy was 4.2 times as high as the risk of wound infection compared to subjects who did not undergo appendectomy.
INCORRECT:
- Subjects taking aspirin had 0.57 less risk of myocardial infarction compared to subjects taking the placebo.
- Subjects who underwent incidental appendectomy had 4.2 times more risk of wound infection compared to subjects who did not undergo appendectomy.
|
Key Concept How to Interpret Risk Ratios: Since the relative risk is a simple ratio, errors tend to occur when the terms "more" or "less" are used. Because it is a ratio and expresses how many times more probable the outcome is in the exposed group, the simplest solution is to incorporate the words "times the risk" or "times as high as" in your interpretation. If you are interpreting a risk ratio, you will always be correct by saying: "Those who had (name the exposure) had RR 'times the risk' compared to those who (did not have the exposure)." Or "The risk of (name the disease) among those who (name the exposure) was RR 'times as high as' the risk of (name the disease) among those who did not (name the exposure)."
|
Question: Does one need to specify the time units for a risk ratio? (Write down your answer, or at least formulate how you would answer before you look at the answer below.)
Rate Ratios
Rate ratios are closely related to risk ratios, but they are computed as the ratio of the incidence rate in an exposed group divided by the incidence rate in an unexposed (or less exposed) comparison group.
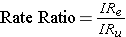
Consider an example from The Nurses' Health Study. This prospective cohort study was used to investigate the effects of hormone replacement therapy (HRT) on coronary artery disease in post-menopausal women. The investigators calculated the incidence rate of coronary artery disease in post-menopausal women who had been taking HRT and compared it to the incidence rate in post-menopausal women who had not taken HRT. The findings are summarized in this table:
|
Post-menopausal Hormone Use |
# with Coronary Artery Disease |
Person-Years of Disease-free Follow-up |
|
Yes |
30 |
54,308.7 |
|
No |
60 |
51,477.5 |
- The rate in those using hormones was 30 / 54,308.7 = 55.2 per 100,000 person-years
- The rate in those NOT using hormones was 60 / 51,477.5 = 116.6 per 100,000 person-years.
So, the rate ratio was 0.47.
Interpretation: Women who used postmenopausal hormones had 0.47 times the rate of coronary artery disease compared to women who did not use postmenopausal hormones.
(Rate ratios are often interpreted as if they were risk ratios, e.g., post-menopausal women using HRT had 0.47 times the risk of CAD compared to women not using HRT, but it is more precise to refer to the ratio of rates rather than risk.)
